Infinitesimal Legos

or, multivariable calculus does manipulatives

May 2023

As a project for our multivariable calculus class, my student Ethan Kim and I designed and 3D-printed a set of infinitesimal Legos: interlocking shapes representing differential areas and volumes in the standard two- and three-dimensional coordinate systems.

There are five in total: one each for rectangular and polar in 2D, and one each for rectangular, spherical, and cylindrical in 3D. Each dimension is labeled with its length (“length”), helping us see how to compute each infinitesimal area/volume (and thus, how to do integrals in those coordinate systems).

The shapes snap together like Legos. That’s mostly for entertainment value, but it does let us see how each coordinate system generalizes (or doesn’t) from dimension to dimension. For instance, a 2D polar differential area can fit beneath (or atop) either a 3D polar or a 3D cylindrical differential volume.

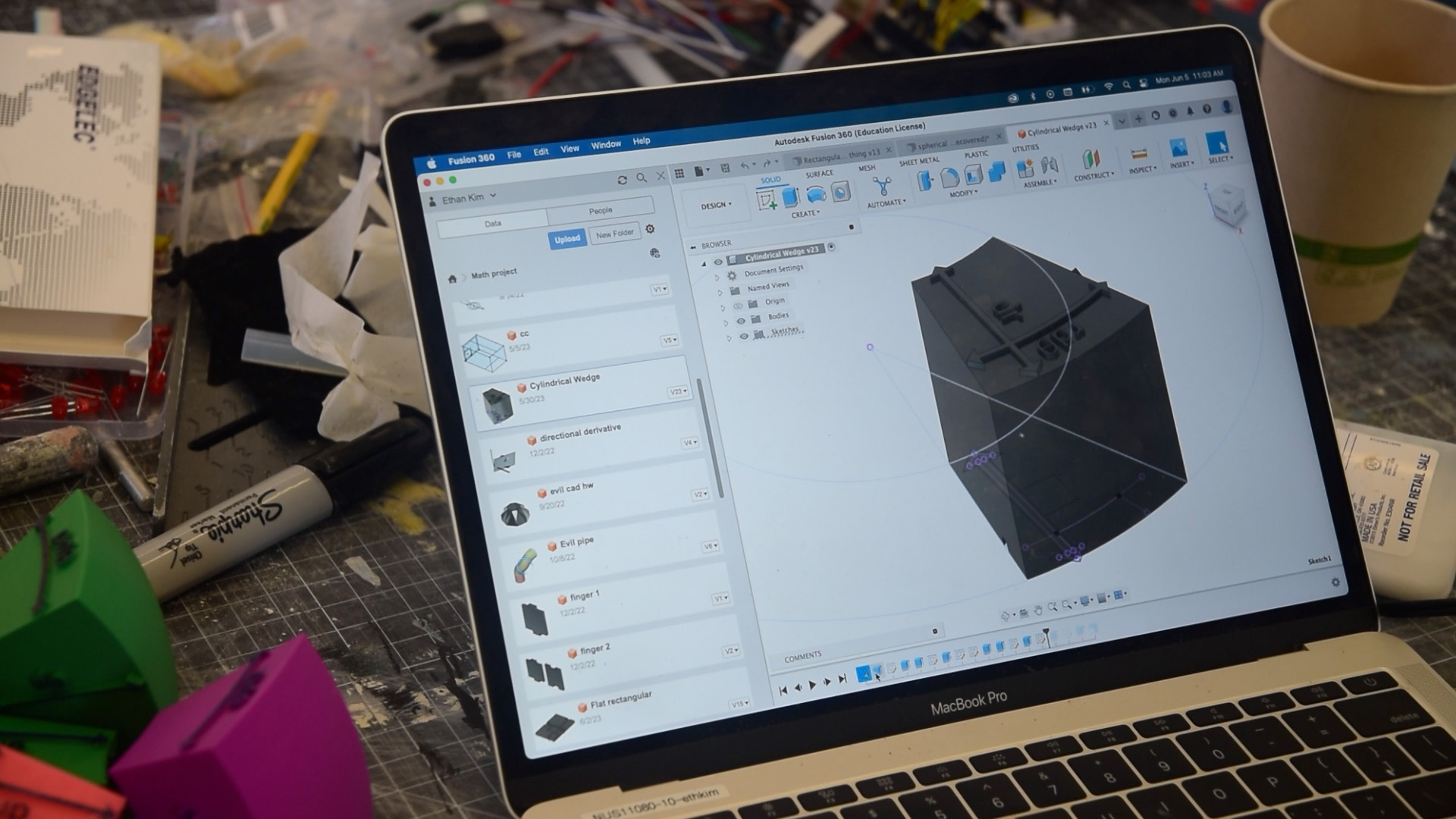
The project grew out of asking the kids to derive what I call the “spherical coordinates correction factor.” One day, as we were starting to work in non-rectangular coordinate systems, I doodled a spherical wedge on the board at the beginning of class, and asked the kids to figure out its volume, using geometry. (The determinant of the derivative matrix and its glories had not yet coalesced in their minds.) Ethan, a leader of the robotics team and now a mechanical engineering undergrad at Princeton, instinctively turned to Fusion360 to CAD a much better spherical wedge than my doodle. “We need to 3D print that!!!,” I said. Quickly this metastasized into a broader vision:

Ethan put up with my endless requests for tweaks over the last few months of school:

After half a dozen revisions, we made a full set of the Legos (including unlabeled ones, for people who don’t yet know the answers!).